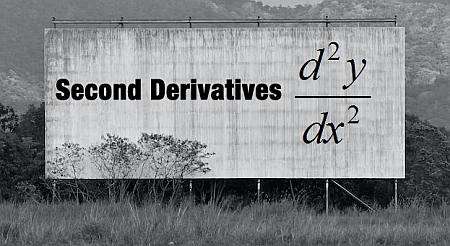求解器微积分

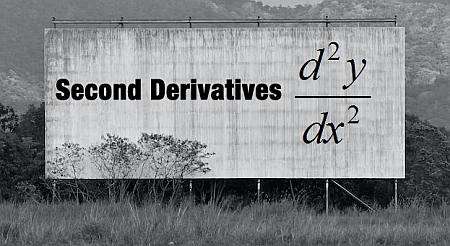
二次导数计算器

指示： 使用二阶导数计算器计算你提供的任何可微调函数的二阶导数（即导数的导数）,显示所有步骤。请在下面的表格框中输入函数。

什么是二阶导数

用非常简单的话来说,二次导数只是导数的导数。因此,计算二次导数的过程包括计算一次导数,然后再计算一次,使用常见的衍生品规则 .一个函数\(f(x)\)的二阶导数通常写成\(f''(x)\)。

二次导数的概念也适用于部分导数 ,它对应于两次导数,但在这种情况下,它可以针对不同的变量进行计算。

计算二阶导数的步骤

步骤1： 确定你要微分两次的函数f(x),并且简化尽可能的先
第2步： 进行一次微分,得到导数f'(x)。如果需要的话,对得到的导数进行简化
第 3 步： 现在对f'(x)进行微分,得到二阶导数f''(x)

这些步骤似乎很容易,但根据给定的函数,在代数计算可能很大。

二次导数记号

二次导数最常用的符号是\(f''(x)\),它很好地反映了一个事实,即用'表示的导数操作被应用于函数的两次。

还有另一种二次导数的符号,当函数\(f(x)\)被称为'y=y(x)'时,这种符号特别有用。那么,我们使用以下符号来表示二阶导数。

\[\displaystyle \frac{d^2y}{dx^2} = \displaystyle \frac{d}{dx} \left(\frac{dy}{dx}\right) \]

计算隐性函数的二阶导数的步骤

步骤1： 找出涉及x和y的方程
第2步： 对等式的两边进行微分。每条边都有可能取决于x,y和y'。简化明显的条款,但这不是严格意义上的需要
第 3 步： 再次对等式的两边进行微分。每条边都有可能取决于x,y,y'和y''。然后,求出y''

如果x和y是由一个方程隐含定义的,如\(x^2 + y^2 = 1\),那么通过隐含微分计算二阶导数通常比先用x求解y然后再进行微分要容易得多。

在某一点的二次导数

与导数一样,二阶导数是一个逐点定义的函数。请注意,学生们常犯的一个错误是认为,既然我想在某一点上微分,而在某一点上评估的函数是常数,那么它的导数一定是常数。这是错误的。你首先计算导数然后你再进行评估。

例子。二次导数计算

计算.的二阶导数。\(f(x) = \cos(x^2)\)的二阶导数

解决方案：在这个例子中,我们将计算函数\(\displaystyle f(x)=\cos\left(x^2\right)\)的二阶导数。

\( \displaystyle \frac{d}{dx}\left(\cos\left(x^2\right)\right)\)

By using the Chain Rule: \(\frac{d}{dx}\left( \cos\left(x^2\right) \right) = \frac{d}{dx}\left(x^2\right)\cdot \left(-\sin\left(x^2\right)\right)\)

\( \displaystyle = \,\,\)

\(\displaystyle \frac{d}{dx}\left(x^2\right)\cdot \left(-\sin\left(x^2\right)\right)\)

We use the Power Rule for polynomial terms: \(\frac{d}{dx}\left( x^2 \right) = 2x\)

\( \displaystyle = \,\,\)

\(\displaystyle \left(2x\right) \left(-\sin\left(x^2\right)\right)\)

which then leads to

\( \displaystyle = \,\,\)

\(\displaystyle 2x\cdot \left(-\sin\left(x^2\right)\right)\)

Finally, the following is obtained

\( \displaystyle = \,\,\)

\(\displaystyle -2x\sin\left(x^2\right)\)

二次派生。 现在,我们对得到的导数进行微分,从而得到第二个导数。

\( \displaystyle \frac{d^2f}{dx^2} = \frac{d}{dx}\left(-2x\sin\left(x^2\right)\right)\)

By using the Product Rule: \(\frac{d}{dx}\left( \left(-1\right)\times 2x\sin\left(x^2\right) \right) = \frac{d}{dx}\left(-2x\right) \cdot \sin\left(x^2\right)+\left(-1\right)\times 2x \cdot \frac{d}{dx}\left(\sin\left(x^2\right)\right)\)

\( \displaystyle = \,\,\)

\(\displaystyle \frac{d}{dx}\left(-2x\right) \cdot \sin\left(x^2\right)+\left(-1\right)\times 2x \cdot \frac{d}{dx}\left(\sin\left(x^2\right)\right)\)

By linearity, we know \(\frac{d}{dx}\left( (-1)\times 2x \right) = \left(-1 \right) \cdot \frac{d}{dx}\left(2x\right)\), so plugging that in:

\( \displaystyle = \,\,\)

\(\displaystyle \left(\left(-1 \right) \cdot \frac{d}{dx}\left(2x\right)\right) \sin\left(x^2\right)+\left(-1\right)\times 2x \cdot \frac{d}{dx}\left(\sin\left(x^2\right)\right)\)

Using the Chain Rule: \(\frac{d}{dx}\left( \sin\left(x^2\right) \right) = \frac{d}{dx}\left(x^2\right)\cdot \cos\left(x^2\right)\) and directly we get: \(\frac{d}{dx}\left( 2x \right) = 2\)

\( \displaystyle = \,\,\)

\(\displaystyle \left(\left(-1 \right) \cdot 2\right) \sin\left(x^2\right)+\left(-1\right)\times 2x \cdot \frac{d}{dx}\left(x^2\right)\cdot \cos\left(x^2\right)\)

In this case we use the Power Rule for polynomial terms: \(\frac{d}{dx}\left( x^2 \right) = 2x\)

\( \displaystyle = \,\,\)

\(\displaystyle \left(\left(-1 \right) \cdot 2\right) \sin\left(x^2\right)+\left(-1\right)\times 2x \cdot 2x\cdot \cos\left(x^2\right)\)

and then we find

\( \displaystyle = \,\,\)

\(\displaystyle -2x\cdot 2x\cos\left(x^2\right)+\left(-2\right)\sin\left(x^2\right)\)

Putting together the numerical values, reducing the ones in \(-2x\cdot 2x\cos\left(x^2\right) = -4x^2\cos\left(x^2\right)\) and grouping the terms with \(x\) in the term \(-2x\cdot 2x\cos\left(x^2\right)\)

\( \displaystyle = \,\,\)

\(\displaystyle -2\cdot 2x^2\cos\left(x^2\right)-2\sin\left(x^2\right)\)

Simplifying the integers that can be multiplied together: \(\displaystyle -2\times2 = -4\)

\( \displaystyle = \,\,\)

\(\displaystyle -4x^2\cos\left(x^2\right)-2\sin\left(x^2\right)\)

最后的结论 :我们发现,我们要找的第二个导数是。

\[f''(x) = -4x^2\cos\left(x^2\right)-2\sin\left(x^2\right)\]

例子。更多二级导数

对于以下函数:\(f(x) = x \cos(x)\),计算其二阶导数

解决方案：现在,我们对tis\(\displaystyle f(x)=x\cos\left(x\right)\)做同样的处理,我们需要计算它的导数。

该函数已经简化,所以我们可以直接计算其导数。

\( \displaystyle \frac{d}{dx}\left(x\cos\left(x\right)\right)\)

Using the Product Rule: \(\frac{d}{dx}\left( x\cos\left(x\right) \right) = \frac{d}{dx}\left(x\right) \cdot \cos\left(x\right)+x \cdot \frac{d}{dx}\left(\cos\left(x\right)\right)\)

\( \displaystyle = \,\,\)

\(\displaystyle \frac{d}{dx}\left(x\right) \cdot \cos\left(x\right)+x \cdot \frac{d}{dx}\left(\cos\left(x\right)\right)\)

Directly differentiating: \(\frac{d}{dx}\left( \cos\left(x\right) \right) = -\sin\left(x\right)\)

\( \displaystyle = \,\,\)

\(\displaystyle \frac{d}{dx}\left(x\right) \cdot \cos\left(x\right)+x \left(-\sin\left(x\right)\right)\)

which then leads to

\( \displaystyle = \,\,\)

\(\displaystyle x\cdot \left(-\sin\left(x\right)\right)+\cos\left(x\right)\)

By reorganizing/simplifying/expanding the terms that are amenable to

\( \displaystyle = \,\,\)

\(\displaystyle -x\sin\left(x\right)+\cos\left(x\right)\)

二次派生计算。 下一步是对前几步中得到的导数进行微分。

\( \displaystyle \frac{d^2f}{dx^2} = \frac{d}{dx}\left(-x\sin\left(x\right)+\cos\left(x\right)\right)\)

By linearity, we know \(\frac{d}{dx}\left( (-1)x\sin(x)+\cos(x) \right) = \frac{d}{dx}\left((-1)x\sin(x)\right)+\frac{d}{dx}\left(\cos(x)\right)\), so plugging that in:

\( \displaystyle = \,\,\)

\(\displaystyle \frac{d}{dx}\left(\left(-1\right)x\sin\left(x\right)\right)+\frac{d}{dx}\left(\cos\left(x\right)\right)\)

Directly differentiating: \(\frac{d}{dx}\left( \cos\left(x\right) \right) = -\sin\left(x\right)\) and we can use the Product Rule: \(\frac{d}{dx}\left( \left(-1\right)x\sin\left(x\right) \right) = \frac{d}{dx}\left(\left(-1\right)x\right) \cdot \sin\left(x\right)+\left(-1\right)x \cdot \frac{d}{dx}\left(\sin\left(x\right)\right)\)

\( \displaystyle = \,\,\)

\(\displaystyle \frac{d}{dx}\left(\left(-1\right)x\right) \cdot \sin\left(x\right)+\left(-1\right)x \cdot \frac{d}{dx}\left(\sin\left(x\right)\right)-\sin\left(x\right)\)

Directly differentiating: \(\frac{d}{dx}\left( \sin\left(x\right) \right) = \cos\left(x\right)\) and directly we get: \(\frac{d}{dx}\left( \left(-1\right)x \right) = -1\)

\( \displaystyle = \,\,\)

\(\displaystyle \left(-1\right) \sin\left(x\right)+\left(-1\right)x \cdot \cos\left(x\right)-\sin\left(x\right)\)

and then we get

\( \displaystyle = \,\,\)

\(\displaystyle \left(-1\right)x\cos\left(x\right)+\left(-1\right)\sin\left(x\right)+\left(-\sin\left(x\right)\right)\)

Reducing the multiplication by ones in \(\left(-1\right)x\cos\left(x\right) = \left(-1\right)x\cos\left(x\right)\) and

\( \displaystyle = \,\,\)

\(\displaystyle \left(-1\right)x\cos\left(x\right)-\sin\left(x\right)+\left(-\sin\left(x\right)\right)\)

Simplifying:

\( \displaystyle = \,\,\)

\(\displaystyle -x\cos\left(x\right)-2\sin\left(x\right)\)

第二个派生结论 :我们得出结论,给定函数的二阶导数是a。

\[f''(x) = -x\cos\left(x\right)-2\sin\left(x\right)\]

例子。二次导数和隐式微分

使用隐式微分法,计算\( x^2 + y^2 = 1\)的y相对于x的二次导数。

解决方案：我们应用隐式微分法,假设y取决于x,我们对等式的两边进行微分。

\[ \frac{d}{dx}\left(x^2 + y^2\right) = \frac{d}{dx} (1) \] \[ \Rightarrow 2x + 2yy' = 0 \]

现在,再次应用隐性微分法。

\[ \frac{d}{dx}\left( 2x + 2yy' \right) = \frac{d}{dx} 0 \] \[ \Rightarrow 2 + 2y'^2+2yy'' = 0 \] \[ \Rightarrow 2y'^2 + 2yy'' = -2\] \[ \Rightarrow yy'' = -1 - y'^2 \] \[ \Rightarrow y'' = \frac{-1 - y'^2}{y} \]

计算结束。