More on this this Right Triangle Calculator

This calculator will allow you calculate a right triangle, along with its perimeter and area, based on the either two sides (legs) or one side (leg) and the hypotenuse.

Once you provide the required information, you need to all you need to do is to click on "Calculate", which will will bring the steps of the calculation to you.

How to Solve a Right Triangle

Completely solving a right triangle using a calculator can absolutely simplify your geometry tasks. Here are the steps you should need to follow in order to do so:

Steps for the calculation of Right Triangle

• Input the known values: In the case of a right triangle, in any case you will need two pieces of information. Indeed, enter the lengths of two sides or one side and an angle.
• The calculation type will be automatically selected: The missing side/hypotenuse will be computed, angle, area, or perimeter.
• Click calculate: The tool will compute the results based on your inputs, step-by-step.
• Review the results: Check the calculated values for accuracy and relevance to your problem, do the solutions make sense. For example, if the calculator provides a negative value for a side, then something must have gone wrong.

Understanding the Pythagorean Theorem

The Pythagorean Theorem is one of the most fundamental tools you have to deal with right triangles. The theorem states that in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides. This can be expressed using the following formula

\[ c^2 = a^2 + b^2 \]

where \( c \) is the hypotenuse, and \( a \) and \( b \) are the other two sides.

Calculating the Sides of a Right Triangle

To calculate the missing side of a right triangle, you can use the Pythagorean theorem if you know two sides. And here is how you do it:

Find the Hypotenuse

• You need to identify the two known sides, \( a \) and \( b \).
• Then, you apply the formula: \( c = \sqrt{a^2 + b^2} \).

Find One of the Other Sides

• Identify the hypotenuse \( c \) and one other side, let's say \( a \).
• Use the formula that comes from the Pythagorean theorem, this time you solve for \(b\) : \( b = \sqrt{c^2 - a^2} \).

How to Find the Missing Side of a Right Triangle?

As we explained in the previous section, the answer depends on which of the sides is missing. Notice that we are using the term "sides" liberally, as in a right triangle you would use hypotenuse to the sides that is opposite to the right angle, and the other sides are referred as generic sides or legs.

The general idea of the approach is that when one side of a right triangle is missing, you can use the Pythagorean theorem or trigonometric functions:

Using Trigonometry

• If you know an angle (not the right angle) and one side in the context of a right triangle, use sine, cosine, or tangent to find the other sides, depending what is the side that is missing.
• For example for a triangle with hypotenuse equal to 2, an angle equal to 30^o = \(\frac{\pi}{6}\), and an opposite side \(s\), we can use the identity involving the cosine function \(\cos(\frac{\pi}{6}) = \frac{s}{2} \), which means that we can solve for \(s\).

Special Right Triangles Explained

Although there are infinitely many right triangles, there are two types of special right triangles with specific properties:

30-60-90 Triangle: Properties and Calculations

This type of right triangle comes from splitting an equilateral triangle in two halves. Therefore, the sides of this type of right triangle are in the ratio \( 1 : \sqrt{3} : 2 \).

If the shortest side is \( x \), then the hypotenuse is \( 2x \), and the other side is \( x\sqrt{3} \), which obtained by using Pythagoras' formula.

The 45-45-90 Triangle: Properties and Calculations

• This type of right triangle occurs when splitting a square into two halves by the diagonal. This triangle is isosceles with sides in the ratio \( 1 : 1 : \sqrt{2} \).
• If one leg is \( x \), the hypotenuse is \( x\sqrt{2} \).

Area and Perimeter of a Right Triangle

Another natural calculation that arises in the case of the right triangle is the calculation of the area and perimeter. To find the area and perimeter of a right triangle:

Area Calculation: We can use the formula: \( \text{Area} = \frac{1}{2} \times \text{base} \times \text{height} \), where in this case the base and height are the two legs (not the hypotenuse).

Perimeter Calculation: For the perimeter, we simply add the lengths of all three sides: \( \text{Perimeter} = a + b + c \).

What is the 3-4-5 Right Triangle Rule?

The 3-4-5 rule is a simple way to verify if a triangle is a right triangle:

• If the sides are in the ratio 3:4:5, the triangle is a right triangle.
• This rule is based on the fact that if the sides are in the 3:4:5 ratio, then the \(a^2 + b^2 = c^2\) rule holds.

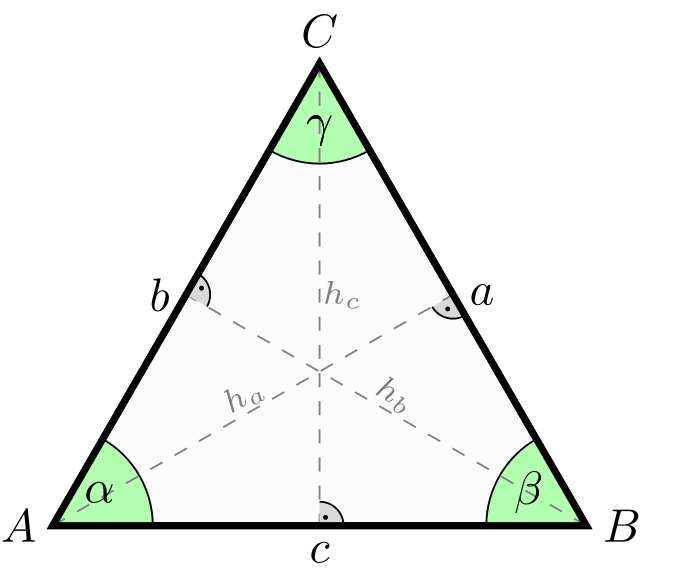
Scalene Triangle Calculator

While not a right triangle, a scalene triangle calculator can help with side lengths and angles:

Calculating Scalene Triangle Properties

• Use the Law of Cosines to find sides or angles.
• Use the Law of Sines for angles when sides are known.

Summary of Right Angle Triangle Formulas

The fundamental formulas for a right triangle include:

• Pythagorean Theorem: \( c^2 = a^2 + b^2 \).
• Area: The formula for the area is \( \text{Area} = \frac{1}{2} \times \text{base} \times \text{height} \).
• Perimeter: The formula is \( \text{Perimeter} = a + b + c \).

Explore More Triangle Calculators

If you're working with triangles and need to explore different scenarios, our site offers several tools that might be useful. For instance, if you know two angles and the side opposite one of them, you can use our Triangle Calculator with two angles and one opposite side to find the remaining sides and angles. This can be particularly handy when dealing with non-right triangles where the Pythagorean theorem doesn't directly apply.

Another useful tool is the Triangle Calculator with three sides known, which is perfect for when you have all the side lengths but need to determine the angles or verify if the triangle is indeed possible. Additionally, for those interested in the classic properties of triangles, our Pythagorean Theorem Calculator can help you confirm or find the length of the hypotenuse in right triangles, ensuring your calculations are accurate.