More About this Normal Distribution Probability Calculator Tool

Here are some facts about the normal distribution probability so you can better understand this normal distribution graph generator. First of all, the normal probability is a type of continuous probability distribution that can take random values on the whole real line. The main properties of the normal distribution are:

• It is continuous (and as a consequence, the probability of getting any single, specific outcome is zero)


• It has a "bell shaped" distribution (and that is where the "Bell-Curve" name comes along)


• The normal distribution is determined by two parameters: the population mean and population standard deviation


• It is symmetric with respect to its mean

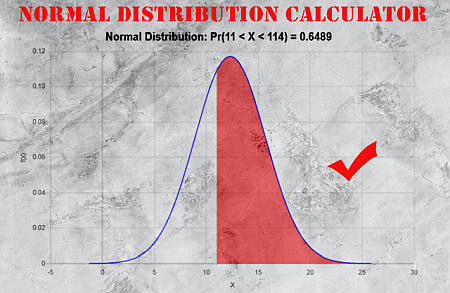
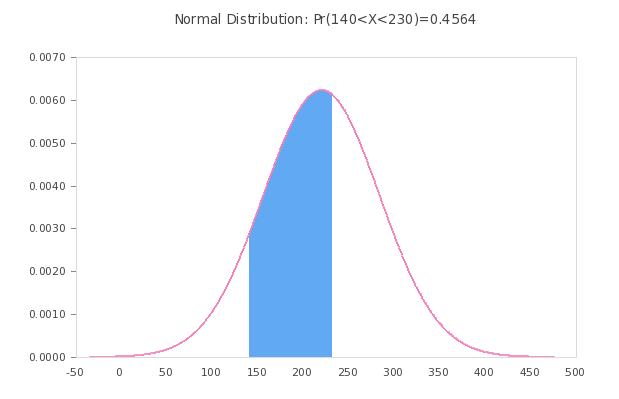
Using the above normal distribution curve calculator , we are able to compute probabilities of the form \(\Pr(a \le X \le b)\), along with its respective normal distribution graphs . This not exactly a normal probability density calculator, but it is a normal distribution (cumulative) calculator. Change the parameters for a and b to graph normal distribution based on your calculation needs. If you need to compute \(\Pr(3 \le X \le 4)\), you will type "3" and "4" in the corresponding boxes of the script.

One very important special case consists of the case of the standard normal distribution , which corresponds to the case of a normal distribution with mean equal to \(\mu = 0\), and standard deviation equal to \(\sigma = 1\). In the cases of a regular normal distribution or of a standard normal distribution can all be handled with the above probability calculator.

Parameters of the Normal Distribution

Therefore, don't forget, the normal distribution is in general determined by its mean and standard deviation. The mean can be any real number and the standard deviation can be any non-negative number. But in particular, the standard normal distribution is a normal distribution that has the property that the mean of the standard normal distribution is zero and the standard deviation of the standard normal distribution is 1.

Please do not hesitate to contact us if you have any question about this normal distribution graph generator.

Other normal distribution calculator

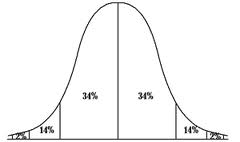
The normal distribution is a crucial continuous distribution. Aside from this probability distribution calculator for normal distributions, you can use the 68-95-99 rule calculator, which shows how to use the of empirical rule.

Also, frequently you are interested in the behavior of sample sizes, for which you can use our sampling distribution calculator, where you are taking the average of samples of size \(n\)

Other continuous distributions

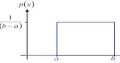
For other type of continuous distributions, you can try for example.our site provides many other continuous distribution calculators such as the exponential distribution calculator . F uniform distribution calculator .