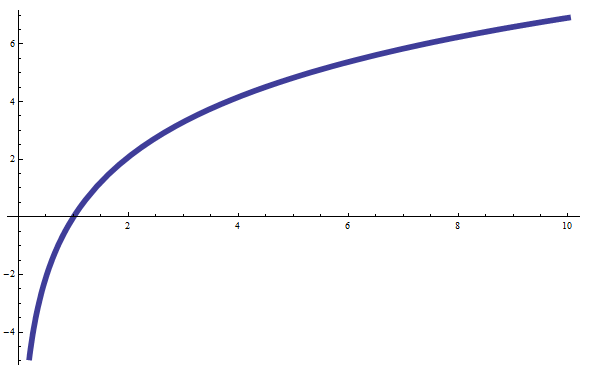
Logarithmic Function Calculator from Two Points

The main purpose of this calculator is to estimate the parameters $A_0$ and $k$ for the logarithmic function $f(t)$ which is defined as:

$$f(t) = A_0 \ln(k t)$$

The parameters need to be so the logarithmic function passes through the two given points $(t_1, y_1)$ and $(t_2, y_2)$.

How do you estimate a logarithmic function from two points?

Algebraically speaking, you need to solve the following system of equations to find the parameters $A_0$ and $k$:

$$y_1 = A_0 \ln(k t_1)$$ $$y_2 = A_0 \ln(k t_2)$$

By solving this system for the unknowns $A_0$ and $k$, we can find unique solutions, as long as $t_1 \ne t_2$.

Indeed, by subtracting both sides of the equations:

$$\displaystyle y_1 - y_2 = A_0 \left( \ln(k t_1) - \ln(k t_2) \right)$$ $$\displaystyle \Rightarrow \, y_1 - y_2 = A_0 \ln \left(\displaystyle\frac{k t_1}{k t_2}\right)$$ $$\displaystyle \Rightarrow \, y_1 - y_2 = A_0 \ln \left(\displaystyle\frac{t_1}{t_2}\right)$$ $$\Rightarrow \, A_0 = \displaystyle \frac{y_1 - y_2}{\ln(t_1) - \ln(t_2)}$$

which solves the equations for $A_0$. Now, in order to solve for $k$ we use the first equation and apply exponential to both sides::

$$y_1 = A_0 \ln(k t_1)$$ $$\Rightarrow \, \displaystyle e^{\frac{y_1}{A_0}} = k t_1$$ $$\Rightarrow \, k = \displaystyle \frac{e^{\frac{y_1}{A_0}}}{t_1}$$

and there we have found $k$, as function of $A_0$ that is already determined and known.

How do you calculate an exponential function?

If instead of a logarithmic function you are interested in exponential behavior, then you should probably use this exponential function calculator , which follows the same logic of estimating parameters to enforce the function passing through two given points.