Logarithmic Function Graph maker
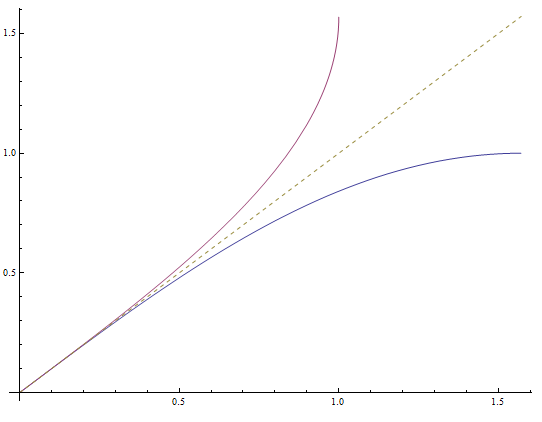
Instructions: This Logarithmic Function Graph maker allows you to plot a logarithmic function, or to compare the graph of two logarithmic functions. You need to provide the base \(b\) of each of the functions \(f(x) = \log_b x\).
Logarithmic Function Graph maker
This graph maker allows you to graph two logarithmic functions, and optionally to evaluate the two functions at several values.
These logarithmic functions that can be graphed with this calculator are:
\[f(x) = \log_b x\]So then, in order to get the corresponding graphs, you just need to specify the bases.
What is the inverse of a logarithmic function and how do you graph it?
The inverse of logarithmic functions are exponential functions . This is roughly speaking. More precisely, the inverse of the function \(f(x) = \log_b x\) is \(f^{-1}(x) = b^x\).
In terms of their graphs, the graph of a log functions and its corresponding exponential inverse are symmetric with respect to the 45 o straight line.
How to graph an exponential function
An exponential function of the form that was specified above will have a characteristic exponential shape, and its general form will depend on whether the rate \(r\) is positive or negative.
For a positive rate \(r\) we will have exponential growth , and for a negative rate \(r\) we will have exponential decay .
What is the use of logarithmic paper?
Logarithmic paper is a special type of paper in which the scale is modified so that now the logarithmic functions are graphed as linear functions. They had their time, nowadays the graphing devices (like this one) are readily available and make logarithmic paper rather obsolete.
How do you estimate a logarithmic function?
Often times, you will want to estimate a logarithmic function to pass through two points , and for that purpose you will be given two points, and you will solve a system to estimate the parameters that are required so that the logarithmic function passes through those points.