More on this this Equilateral Triangle Calculator

This calculator will allow you to solve an equilateral triangle, given that you provide the length of a side \(s\), the height \(h\), or its area \(A\), which ever is known. You DO need to provide one of those in order for the calculations to be conducted.

Once you provide valid information (a side, height or area, in the form of a valid numeric expression that is positive), \ the next step is to press the "Calculate" button, to get provided with the steps of the calculations for the triangle.

What is an Equilateral Triangle?

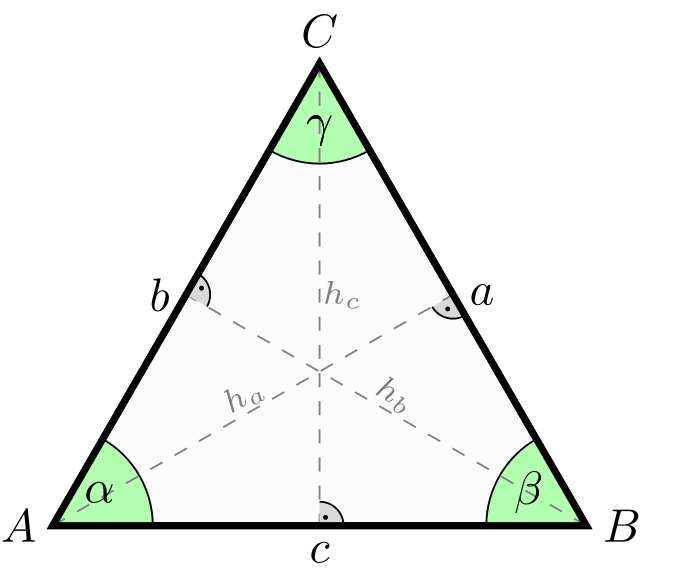
An equilateral triangle is a special type of triangle where all three sides are of equal length, and all internal angles measure 60 degrees, which corresponds to \(\pi/3\) radians. This type of symmetry makes it unique among all possible triangles, offering simplicity in calculations and a pleasing aesthetic in geometric designs, holding a special place in Geometry.

Their original dates back to the ancient Greeks, to Euclid, who gave a formal definition of it in his book Elements, although his original construction is known to be wrong.

How to Use an Equilateral Triangle Calculator?

Using an equilateral triangle calculator can simplify your geometry tasks, especially those relative to computing an equilateral triangle's height, perimeter and area. Here are the steps you should follow:

Steps to Use an Equilateral Triangle Calculator

• Enter the known value corresponding to the side's length
• Alternatively, an equilateral could be solved from its area, perimeter or height
• Click on 'Calculate' to get the results you want.

Equilateral Triangle Formula: Area, Perimeter, and Height

Here are the fundamental formulas for an equilateral triangle, which are simply derived using Pythagorean Theorem:

• Height: \( h = \frac{\sqrt{3}}{2} \times a \). This obtained by using Pythagoras theorem for the right triangle formed by the hypotenuse \(a\) and the half-side formed by the altitude \(a\2\).
• Area: \( A = \frac{\sqrt{3}}{4} \times a^2 \), where \( a \) is the side length, which is simple the result of doing Area = \(\frac{1}{2}\) base x height.
• Perimeter: \( P = 3a \). This one comes directly by

Calculating the Side Length of an Equilateral Triangle

The reverse process can be also be accomplished: If you know the area (A )or height (h) of an equilateral triangle, you can find the side length using these formulas:

• From Area: \( a = \sqrt{\frac{4A}{\sqrt{3}}} \).
• From Height: \( a = \frac{2h}{\sqrt{3}} \).

Though the most common process consists of having the length of a side \(a\) and work our way around from that, the other way around is not completely unusual, and it is worth to be aware of it.

Equilateral Triangle Properties

Equilateral triangles, due to their strong symmetry, have several unique properties:

• All sides are equal.
• All angles are 60 degrees, or \(\frac{\pi}{3}\) if using radians.
• The centroid, circumcenter (the point where the three perpendicular bisectors intersect), incenter (the point where the three angle bisectors intersect), and orthocenter (the point where the three altitudes intersect) coincide at the same point.
• It is the only regular polygon whose interior angles are all integer multiples of exterior angles.

How Do You Find the Value of an Equilateral Triangle?

That question could be considered as a bit vague. The "value" of an equilateral triangle can refer to different properties like area, side length, or height. Here's how you can find all the elements of interest:

• Area: Use the formula \( A = \frac{\sqrt{3}}{4} \times a^2 \).
• Side Length: If you know the area, use \( a = \sqrt{\frac{4A}{\sqrt{3}}} \).
• Height: Use \( h = \frac{\sqrt{3}}{2} \times a \).

Is an Equilateral Triangle a 30-60-90 Triangle?

No, an equilateral triangle is not a 30-60-90 triangle. A 30-60-90 triangle has angles of 30°, 60°, and 90°, whereas an equilateral triangle has all angles equal to 60°. Yet, a 30-60-90 triangle is noteworthy as well, because it corresponds to an isosceles triangle, which has still a solid degree of symmetry.

Height of Equilateral Triangle Calculator

Why is the calculation of the height so important? That is because the height is crucial at the time of computing the area of the triangle. To calculate the height of an equilateral triangle, as we have mentioned it before, you need to use the formula:

\[ h = \frac{\sqrt{3}}{2} \times a \]

where \( a \) is the side length. Where does the \(\sqrt 3\) comes from? When using Pythagoras, we compute \(h^2 = a^2 - \left(\frac{a}{2}\right)^2 = a^2 - \frac{a^2}{4}= \frac{3a^2}{4}\)

Scalene Triangle vs. Equilateral Triangle

In what way does an scalene triangle differs from an equilateral triangle? A scalene triangle has all sides of different lengths, while an equilateral triangle has all sides equal. Here are some key differences:

• Scalene Triangle: No equal sides or angles.
• Equilateral Triangle: All sides and angles are equal.

Notice that a triangle can be neither scalene nor equilateral, situation that happens with an isosceles triangle.

FAQs on Equilateral Triangles

What is the formula for an equilateral triangle?

The formula for the area of an equilateral triangle is \( A = \frac{\sqrt{3}}{4} \times a^2 \).

How to find sides of an equilateral triangle?

If you know the area, use \( a = \sqrt{\frac{4A}{\sqrt{3}}} \). If you know the height, use \( a = \frac{2h}{\sqrt{3}} \).

How do you find the value of an equilateral triangle?

The "value" can refer to different properties. Use the formulas provided for area, side length, or height.

Is a triangle with 30-60-90 equilateral?

No, an equilateral triangle has all angles equal to 60°, not 30-60-90.

Explore More Triangle Calculators

If you're dealing with triangles that are not equilateral, our Triangle Calculator with two angles and one opposite side can be particularly useful. This tool helps you solve for the remaining sides and angles when you only know two angles and the length of the side opposite one of them.

Additionally, if you have all three sides of a triangle known, our Triangle Calculator with three sides known will quickly compute the angles for you, providing a comprehensive understanding of any triangle's geometry.

When working with triangles, understanding the relationships between sides and angles is crucial. For those scenarios where you know two sides and the angle between them, our Triangle Calculator for Two Sides Known and the Angle between them can help you find the remaining side and angles.

Moreover, if you're exploring the fundamental principles of triangle geometry, our Pythagorean Theorem Calculator can assist in verifying or calculating the lengths of the sides in right triangles, ensuring your calculations are accurate.