How to Use this Critical Correlation Calculator

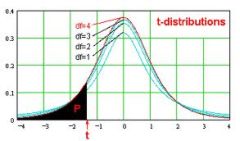
The significance of a sample correlation coefficient \(r\) is tested using the following t-statistic:

\[t = r \sqrt{\frac{n-2}{1-r^2}}\]

For a given sample size \(n\), the number of degrees of freedom is \(df = n-2\), and then, a critical t-value for the given significance level \(\alpha\) and \(df\) can be found. Let us call this critical t-value \(t_c\). Using the expression of the t-statistic:

\[t_c = r \sqrt{\frac{n-2}{1-r^2}} = r \sqrt{\frac{df}{1-r^2}}\]

and now if we solve for \(r\) we find that

\[r_c = \sqrt{\frac{\frac{t_c^2}{df}}{\frac{t_c^2}{df}+1}}\]

and this value of \(r_c\) is the so called critical correlation value used to assess the significance of the sample correlation coefficient \(r\). These critical correlation values are usually found in specific tables.

Observe that this calculator applies for Pearson's correlation, so you would need to use a Spearman’s Critical Correlation Calculator if you are dealing with Spearman's correlation coefficient.

If you have sample data and you want to compute the correlation coefficient, please use our correlation coefficient calculator . If you have many variables, you can also use our correlation matrix calculator .