How to Use this Critical Correlation Calculator

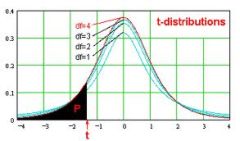
The significance of a sample correlation coefficient $r$ is tested using the following t-statistic:

$$t = r \sqrt{\frac{n-2}{1-r^2}}$$

For a given sample size $n$, the number of degrees of freedom is $df = n-2$, and then, a critical t-value for the given significance level $\alpha$ and $df$ can be found. Let us call this critical t-value $t_c$. Using the expression of the t-statistic:

$$t_c = r \sqrt{\frac{n-2}{1-r^2}} = r \sqrt{\frac{df}{1-r^2}}$$

and now if we solve for $r$ we find that

$$r_c = \sqrt{\frac{\frac{t_c^2}{df}}{\frac{t_c^2}{df}+1}}$$

and this value of $r_c$ is the so called critical correlation value used to assess the significance of the sample correlation coefficient $r$. These critical correlation values are usually found in specific tables.

Observe that this calculator applies for Pearson's correlation, so you would need to use a Spearman’s Critical Correlation Calculator if you are dealing with Spearman's correlation coefficient.

If you have sample data and you want to compute the correlation coefficient, please use our correlation coefficient calculator . If you have many variables, you can also use our correlation matrix calculator .