Residual Plot Grapher
Instructions: Use this Residual Plot Grapher to construct a residual plot for the value obtained with a linear regression analys based on the sample data provided by you. Please input the data for the independent variable and the dependent variable (), in the form below:
About this Residual Plot Grapher
One of the main requirements for the results and predictions from a regression analysis to be valid is for the linear regression assumptions to be met. The assumptions of independence, normality and homoskedasticity of errors is crucial for having reliable regression results
The use of plots based on residuals is crucial to quick assess whether or not the assumptions not met, and whether a correction is needed.
Once we have estimate the regression coefficients corresponding to the y-intercept and slope, and , we can proceed with the calculation of predicted values.
How do you compute regression residual values?
The calculation is simple. The first step consist of computing the linear regression coefficients, which are used in the following way to compute the predicted values:
Once the predicted values are calculated, we can compute the residuals as follows:
What does a residual plot show you?
Residual plots are used to verify linear regression assumptions. It is a visual way to quickly assess whether the assumptions are severely violated or not. For a more concise assessment of the fulfillment of the linear regression assumptions, there are specific statistics test for each assumption.
The different types of residual plots are: residuals versus observation number (provided by this calculator), which is used to assess the hypothesis of independence of error. Also, we have the normality plot of residuals (which is used to assess the normality of errors) and the residuals versus predicted value plot, which is used to assess the assumption of homoskedasticity of error.
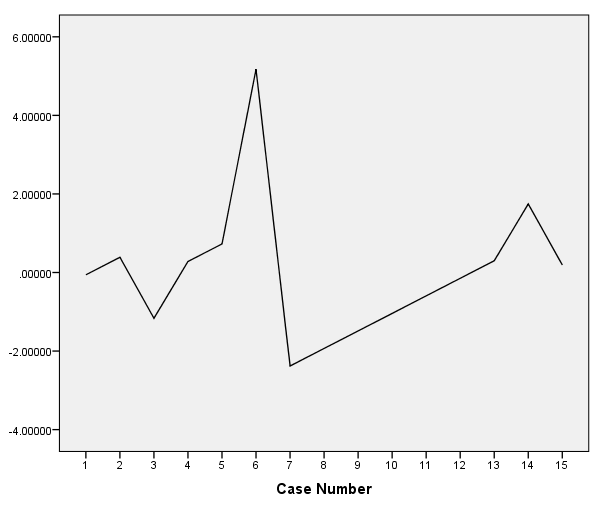
How do you graph residuals from a linear regression model?
There are different types of plots involving residuals. This calculator will show you the calculation of residuals and it will show you a graph of residuals versus observation number.
This residual plot maker allows you to assess whether or not the residuals seem of appear randomly in time (so they are independent), or whether there is some sort of pattern in time (which would indicate that the residuals would not be independent, and a regression assumption would be violated).
If you only need to compute regression results, you can use this linear regression model calculator , in order to get the estimated regression coefficients based on the sample data provided.