How Do You Compute a z-score from a Percentile?

As you know, the Z-scores are normalized scores that serve the purpose of taking scores of a generic normal distribution into equivalent scores in the standard normal distribution (equivalent in the sense of their location relative to their population). Z-scores have numerous applications, the most practical of them being that of being able to compare scores that come from different normal distributions. This comparison is done by normalizing the scores that come from different normal distributions and normalizing them. Then the comparison is made by comparing the corresponding z-scores

Relationship between percentiles and z-scores

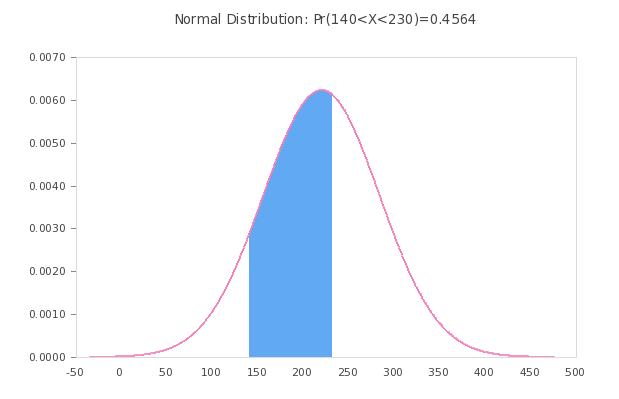
For a given percentile \(p\), which is a number between 0-1, finding the corresponding z-score is done by finding the value of \(z^*\) that solves the following:

\[ p = \Pr(Z < z^*) \]

How do we find such \(z^*\)? The idea is to a percentile to z score conversion table, which is essentially using a standard normal distribution table. This can also be achieved by using Excel.

If conversely what you have is a z-score, you can use our z-score to percentile calculator . Or you may be interested in computing general normal probabilities . Or also, you may have a z-score and you want to compute the corresponding associated raw score, for which you can compute our raw score calculator.

Another task you may be interested in conducting is to compute Percentile Ranks for which you can use this calculator

What if we know the mean and standard deviation?

If you know the mean and standard deviation and you know that the distribution is normal, you can use the this percentile calculator with mean and standard deviation , in which case you get an exact percentile, not an approximation like it is the case with sample data.