More about this Half Life Calculator

The idea behind the concept of half life is to find out how long it takes for a function decrease its value by half.

This concept is strongly motivated by radioactive decay , in which radioactive material decays exponentially, and there is the property that for each specific radioactive material, its content gets reduced by half every certain number of years. The period of time is the half life

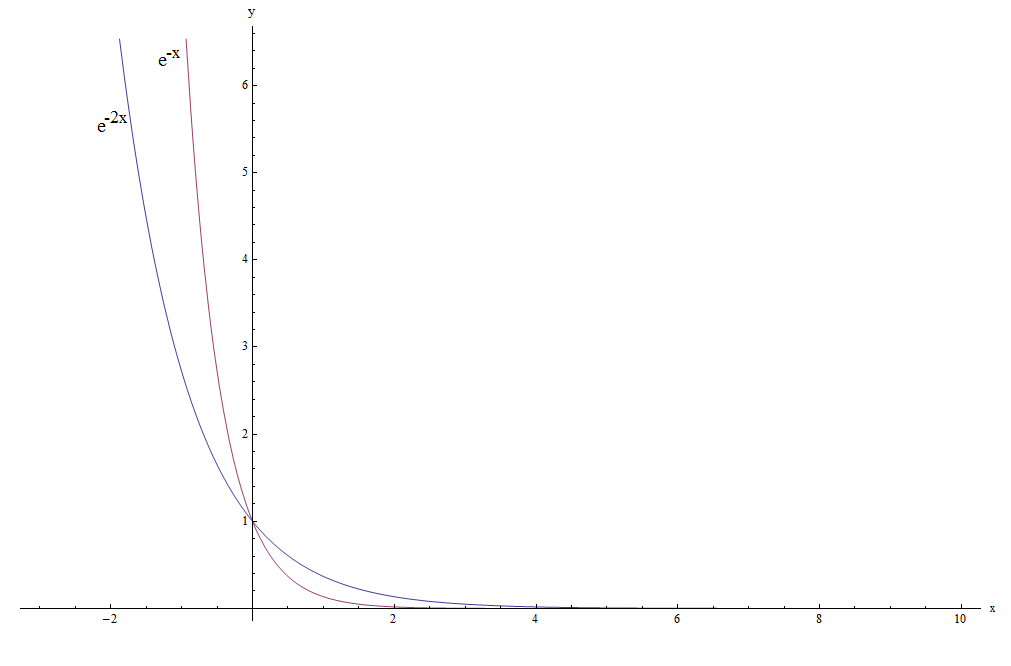
In general, if we consider an exponential decay function:

\[f(t) = A_0 b^{-kt}\]

we want can see that \(f(0) = A_0\), and we want to find \(h\) so that \(f(h) = A_0/2\). To that end, we notice that

\[\displaystyle \frac{A_0}{2}= f(h) = A_0 b^{-kh}\] \[\Rightarrow \displaystyle \frac{1}{2}= b^{-kh}\] \[\Rightarrow \displaystyle \ln\left(\frac{1}{2} = \ln\left(b^{-kh}\right)\] \[\Rightarrow \displaystyle -\ln 2 = -kh \ln b\]\ \[\Rightarrow \displaystyle h = \frac{\ln 2}{k\ln b}\]

What if you have to find the exponential function from two points it passes through?

In that case, we would need to solve:

\[y_1 = A_0 b^{-kt_1}\] \[y_2 = A_0 b^{-kt_2}\]

and to solve for \(A\) and \(k\), and then directly apply the above formula for finding the half-life \(h\).

How do you calculate half life?

The half-life is computed by algebraically finding how time it takes for a function to decrease by half, as it was shown in the section above. For majority of functions, the amount of time required for the function to decrease by half depends on the starting point.

But for functions with exponential decay, the time the function takes to reduce its value by half is independent of the starting point.

How do you calculate decay using half life?

Naturally, decay rate and an exponential decay function itself are tightly related to the half life. Indeed, assume that the half-life \(h\) is known, and \(A_0\) is the initial amount (at \(t = 0\)). Then, the exponential decay function can be written as follows:

\[f(t) = A_0 \cdot 2^{-t/h}\]