Z-test for two Means, with Known Population Standard Deviations
Instructions: This calculator conducts a Z-test for two population means (\(\mu_1\) and \(\mu_2\)), with known population standard deviations ( \(\sigma_1\) and \(\sigma_2\)). Please select the null and alternative hypotheses, type the significance level, the sample means, the population standard deviations, the sample sizes, and the results of the z-test will be displayed for you:
The Z-test for Two Means
More about the z-test for two means so you can better use the results delivered by this solver: A z-test for two means is a hypothesis test that attempts to make a claim about the population means (\(\mu_1\) and \(\mu_2\)). More specifically, we are interested in assessing whether or not it is reasonable to claim that the two population means the population means \(\mu\) 1 and \(\mu\) 2 are equal, based on the information provided by the samples. The test has two non-overlapping hypotheses, the null and the alternative hypothesis.
The null hypothesis is a statement about the population means, corresponding to the assumption of no effect, and the alternative hypothesis is the complementary hypothesis to the null hypothesis. The main properties of a one sample z-test for two population means are:
- Depending on our knowledge about the "no effect" situation, the z-test can be two-tailed, left-tailed or right-tailed
- The main principle of hypothesis testing is that the null hypothesis is rejected if the test statistic obtained is sufficiently unlikely under the assumption that the null hypothesis is true
- The p-value is the probability of obtaining sample results as extreme or more extreme than the sample results obtained, under the assumption that the null hypothesis is true
- In a hypothesis tests there are two types of errors. Type I error occurs when we reject a true null hypothesis, and the Type II error occurs when we fail to reject a false null hypothesis
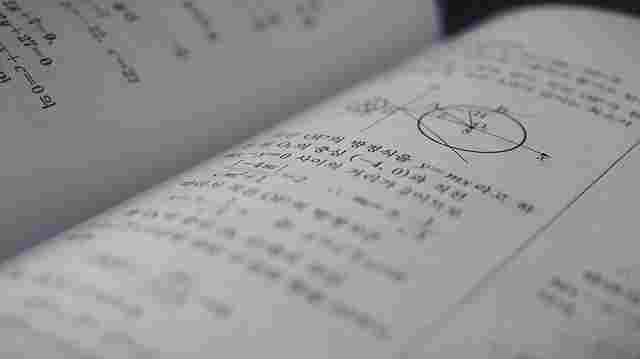
How to calculate the test statistic for the two samples? We have that the formula for a z-statistic for two population means is:
\[z = \displaystyle{\frac{\bar X_1 - \bar X_2}{\sqrt{\displaystyle{\frac{\sigma_1^2}{n_1}} + \displaystyle{\frac{\sigma_2^2}{n_2}} }}} \]The above formula allows you to assess whether or not there is a statistically significant difference between two means. The null hypothesis is rejected when the z-statistic lies on the rejection region, which is determined by the significance level (\(\alpha\)) and the type of tail (two-tailed, left-tailed or right-tailed).
In case that the population standard deviations are not known, you can use a t-test for two sample means calculator .
