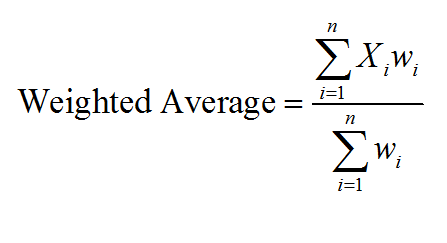[Step-by-Step] A circle is inscribed in a square as shown in the figure: The circumference of the circle is increasing at a constant rate of 6 inches per
Question: A circle is inscribed in a square as shown in the figure:
The circumference of the circle is increasing at a constant rate of 6 inches per second. As the circle, the square expands to maintain the condition of tangency
- Find the rate at which perimeter of the square is increasing
- At the instant when the area of the circle is in 2 , find the rate of increase in the area enclosed between the circle and the square.
Solution:

Price: $2.99
Solution: The downloadable solution consists of 2 pages
Deliverable: Word Document
Deliverable: Word Document

I agree with the Terms & Conditions
All sales are final.

![[Step-by-Step] The graph of a differentiable function f on the [Step-by-Step] The graph of a differentiable function](/images/solutions/MC-solution-library-32876.jpg)
![[Step-by-Step] A breeder of thoroughbred horses wishes to model [Step-by-Step] A breeder of thoroughbred horses wishes](/images/solutions/MC-solution-library-32877.jpg)
![[All Steps] We have FACTOR B Level 1 2 3 FACTOR A 1 4.0, 4.2 5.0, [All Steps] We have FACTOR B Level](/images/solutions/MC-solution-library-32878.jpg)

![[Solution] We are interested in comparing the avg. supermarket [Solution] We are interested in comparing the](/images/solutions/MC-solution-library-32880.jpg)