GP Unit 4

Name:

Section Number:

Instructions:

• Identify the document by typing your full name and section number next to the yellow text.
• Rename the file by adding your last names to current file name (e.g., "u4gp_lastnames.doc").
• Type your answers next to the yellow text.
• Answers requiring decimal places should be rounded to two decimals. This includes rounding dollar and cent amounts to the nearest cent.
• To show your work, you will need to include

o the formula with substituted values.

o the final calculated answer with units.

· To utilize the scientific calculator on your computer, do the following:

o Open the calculator (if it is not in the accessories folder, then select Run from the Start menu)

o Select View from the drop down menu

o Select Scientific to utilize the calculator.

o Note that x^y computes any number to any power (integer, fraction, decimal).

Please add your file.

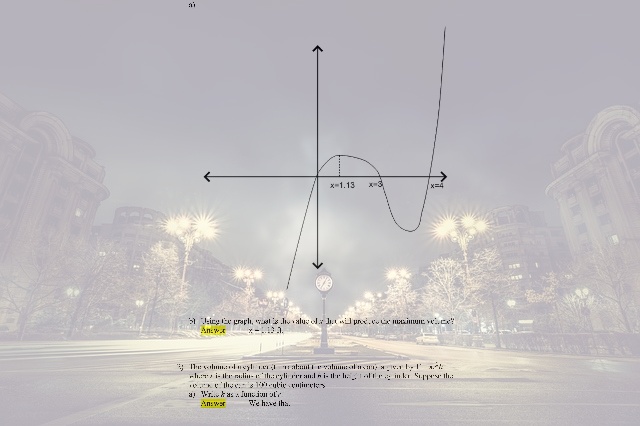
1) An open-top box is to be constructed from a 6 by 8 foot rectangular cardboard by cutting out equal squares at each corner and the folding up the flaps. Let x denote the length of each side of the square to be cut out.

a) Find the function V that represents the volume of the box in terms of x.

Graph this function.

b) Using the graph, what is the value of x that will produce the maximum volume?

2) The volume of a cylinder (think about the volume of a can) is given by V = πr²h where r is the radius of the cylinder and h is the height of the cylinder. Suppose the volume of the can is 100 cubic centimeters.

a) Write h as a function of r.

b) What is the measurement of the height if the radius of the cylinder is 2 centimeters?

c) Graph this function.

3) The formula for calculating the amount of money returned for an initial deposit money into a bank account or CD (Certificate of Deposit) is given by

\[A=P{{\left( 1+\frac{r}{n} \right)}^{nt}}\]

4)
A is the amount of returned.
P is the principal amount initially deposited.
r is the annual interest rate (expressed as a decimal).
n is the compound period.
t is the number of years.

Suppose you deposit $10,000 for 2 years at a rate of 10%.

a) Calculate the return (A) if the bank compounds annually (n = 1).

b) Calculate the return (A) if the bank compounds quarterly (n = 4).

c) Calculate the return (A) if the bank compounds monthly (n = 12).

d) Calculate the return (A) if the bank compounds daily (n = 365).

e) What observation can you make about the increase in your return as your compounding increases more frequently?

f) If a bank compounds continuous, then the formula takes a simpler form, that is
\[A=P{{e}^{rt}}\]

where e is a constant and equals approximately 2.7183.
Calculate A with continuous compounding.

g) Now suppose, instead of knowing t, we know that the bank returned to us $15,000 with the bank compounding continuously. Using logarithms, find how long we left the money in the bank (find t).

h) A commonly asked question is, “How long will it take to double my money?” At 10% interest rate and continuous compounding, what is the answer?

5) For a fixed rate, a fixed principal amount, and a fixed compounding cycle, the return is an exponential function of time. Using the formula, \(A=P{{\left( 1+\frac{r}{n} \right)}^{nt}}\), let r = 10%, P = 1, and n = 1 and give the coordinates (t, A) for the points where t = 0, 1, 2, 3, 4.

a) Show coordinates in this space

Price: $36.95

Solution: The downloadable solution consists of 9 pages, and 914 words
Deliverable: Word Document and pdf