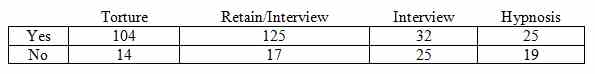
Chi-Square test for One Population Variance

More about the Chi-Square test for one variance so you can better understand the results provided by this solver: A Chi-Square test for one population variance is a hypothesis that attempts to make a claim about the population variance ($\sigma^2$) based on sample information.

Main Properties of the Chi-Square Distribution

The test, as every other well formed hypothesis test, has two non-overlapping hypotheses, the null and the alternative hypothesis. The null hypothesis is a statement about the population variance which represents the assumption of no effect, and the alternative hypothesis is the complementary hypothesis to the null hypothesis.

The main properties of a one sample Chi-Square test for one population variance are:

• The distribution of the test statistic is the Chi-Square distribution, with n-1 degrees of freedom


• The Chi-Square distribution is one of the most important distributions in statistics, together with the normal distribution and the F-distribution


• Depending on our knowledge about the "no effect" situation, the Chi-Square test can be two-tailed, left-tailed or right-tailed


• The main principle of hypothesis testing is that the null hypothesis is rejected if the test statistic obtained is sufficiently unlikely under the assumption that the null hypothesis is true


• The p-value is the probability of obtaining sample results as extreme or more extreme than the sample results obtained, under the assumption that the null hypothesis is true


• In a hypothesis tests there are two types of errors. Type I error occurs when we reject a true null hypothesis, and the Type II error occurs when we fail to reject a false null hypothesis

Can you use Chi-square for one variable?

Absolutely! The Chi-Square statistics is a very versatile statistics, that can be used for a one-way situation (one variable) for example for testing for one variance, or for a goodness of fit test.

But it can also be used for a two-way situation (two variables) for example for a Chi-Square test of independence.

How do you do hypothesis test for single population variance?

The sample variance $s^2$ has some very interesting distributional properties. In fact, based on how the variance is constructed, we can think of the variance as the sum of pieces that have a standard normal distribution but they are squared.

Without getting into much detail, the sum of squared standard normal distributions is tightly related to the Chi-Square distribution, as we will see in the next section.

What is the Chi-Square Formula?

The formula for a Chi-Square statistic for testing for one population variance is

$$\chi^2 = \frac{(n-1)s^2}{\sigma^2}$$

The null hypothesis is rejected when the Chi-Square statistic lies on the rejection region, which is determined by the significance level ($\alpha$) and the type of tail (two-tailed, left-tailed or right-tailed).

To compute critical values directly, please go to our Chi-Square critical values calculator